Bc Review Worksheet
ADVERTISEMENT
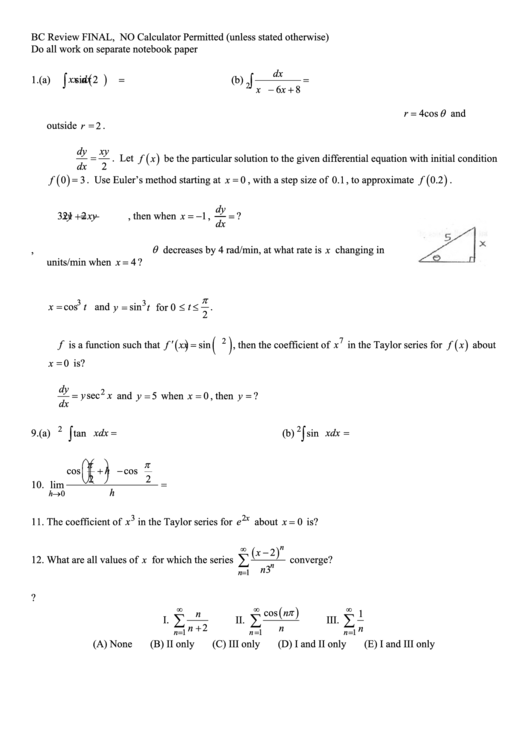
BC Review FINAL, NO Calculator Permitted (unless stated otherwise)
Do all work on separate notebook paper
dx
x dx
1. (a)
x
sin 2
(b)
2
x
6
x
8
2. Write an integral expression which gives the area of the region inside the polar curve
r
4 cos
and
r .
outside
2
dy
xy
3. Given
. Let
f x be the particular solution to the given differential equation with initial condition
dx
2
. Use Euler’s method starting at
x , with a step size of 0.1 , to approximate
f
0
3
0
f
0.2
.
dy
?
x ,
4. If 3
xy
2
x
1 2
y
, then when
1
dx
5. If in the triangle at right, decreases by 4 rad/min, at what rate is x changing in
x ?
units/min when
4
6. Write an integral equation which gives the length of the path described by the parametric equations
3
3
x
cos
t
and
for 0
t
.
y
sin
t
2
2
7
x in the Taylor series for
7. If f is a function such that
f x
sin
x
, then the coefficient of
f x about
x
0
is?
dy
2
y
x , then y ?
y
sec
x
8. If
and
5
when
0
dx
2
2
tan xdx
sin xdx
9. (a)
(b)
cos
h
cos
2
2
10.
lim
h
h
0
3
2x
x
11. The coefficient of
x in the Taylor series for
e
about
0
is?
n
x
2
12. What are all values of x for which the series
converge?
n
n
3
n
1
13. Which of the following converge?
cos
n
n
1
I.
II.
III.
n
2
n
n
n
1
n
1
n
1
(A) None
(B) II only
(C) III only
(D) I and II only
(E) I and III only
ADVERTISEMENT
0 votes
Related Articles
Related forms
Related Categories
Parent category: Education
 1
1 2
2