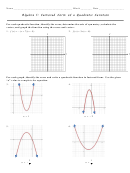
Determining The Number Of X-Intercepts Of The Graph Of A Quadratic Function Worksheet printable pdf download

Determining The Number Of X-Intercepts Of The Graph Of A Quadratic Function Worksheet

CA Standard Alg 1 22.0

Lesson 23

Determining the Number of

x-Intercepts of the Graph

of a Quadratic Function

The number of roots of a quadratic function corresponds to the number of

times the graph of a quadratic function intersects the x-axis. The graph of a

quadratic function can cross the x-axis at two points, one point, or no points

at all. Without graphing a function, you can use factoring or the quadratic

formula to determine the number of x-intercepts of that function.

Use Factoring to Find the Number of x-Intercepts of a

Quadratic Function

The standard form of a quadratic function is y

c, where a, b,

and c are constants. The graph of the function intersects the x-axis when y

equals 0. If the function can be factored, then there are one or two roots.

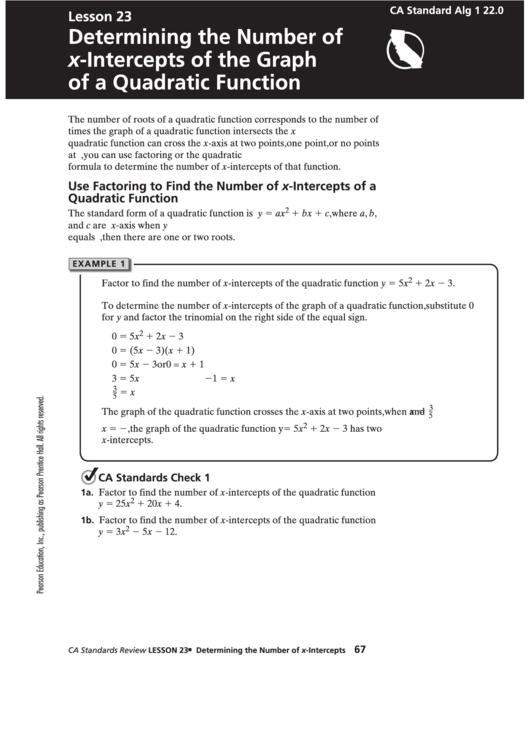
EXAMPLE 1

Factor to find the number of x-intercepts of the quadratic function y

To determine the number of x-intercepts of the graph of a quadratic function, substitute 0

for y and factor the trinomial on the right side of the equal sign.

(5x

3)(x

0 = x

x 5

The graph of the quadratic function crosses the x-axis at two points, when

and

1. Therefore, the graph of the quadratic function y

3 has two

x-intercepts.

CA Standards Check 1

1a. Factor to find the number of x-intercepts of the quadratic function

25x

20x

1b. Factor to find the number of x-intercepts of the quadratic function

12.

CA Standards Review

LESSON 23

■

Determining the Number of x-Intercepts

Determining The Number Of X-Intercepts Of The Graph Of A Quadratic Function Worksheet