Ap Calculus 2 - Differential Equations And Exponential Growth
ADVERTISEMENT
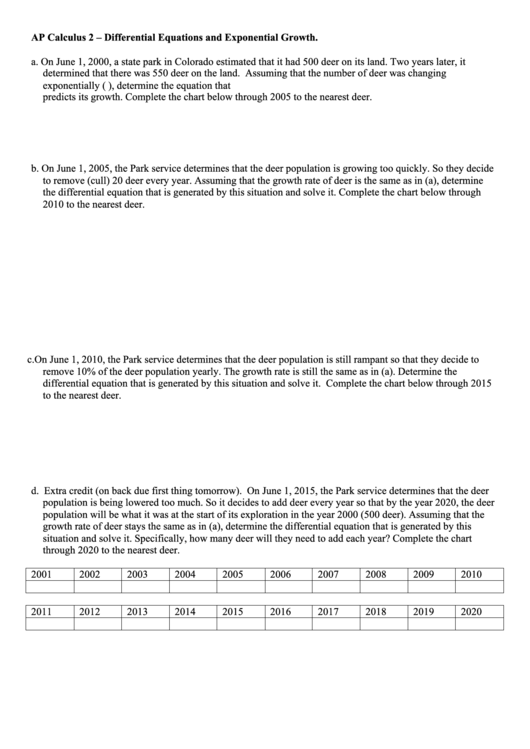
AP Calculus 2 – Differential Equations and Exponential Growth.
a. On June 1, 2000, a state park in Colorado estimated that it had 500 deer on its land. Two years later, it
determined that there was 550 deer on the land. Assuming that the number of deer was changing
exponentially (i.e. its rate of change was proportional to the current amount), determine the equation that
predicts its growth. Complete the chart below through 2005 to the nearest deer.
b. On June 1, 2005, the Park service determines that the deer population is growing too quickly. So they decide
to remove (cull) 20 deer every year. Assuming that the growth rate of deer is the same as in (a), determine
the differential equation that is generated by this situation and solve it. Complete the chart below through
2010 to the nearest deer.
c. On June 1, 2010, the Park service determines that the deer population is still rampant so that they decide to
remove 10% of the deer population yearly. The growth rate is still the same as in (a). Determine the
differential equation that is generated by this situation and solve it. Complete the chart below through 2015
to the nearest deer.
d. Extra credit (on back due first thing tomorrow). On June 1, 2015, the Park service determines that the deer
population is being lowered too much. So it decides to add deer every year so that by the year 2020, the deer
population will be what it was at the start of its exploration in the year 2000 (500 deer). Assuming that the
growth rate of deer stays the same as in (a), determine the differential equation that is generated by this
situation and solve it. Specifically, how many deer will they need to add each year? Complete the chart
through 2020 to the nearest deer.
2001
2002
2003
2004
2005
2006
2007
2008
2009
2010
2011
2012
2013
2014
2015
2016
2017
2018
2019
2020
Stu Schwartz
ADVERTISEMENT
0 votes
Related Articles
Related forms
Related Categories
Parent category: Life
 1
1 2
2 3
3








