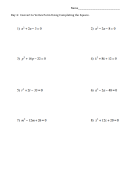Completing The Square Using Algebra Tiles Worksheet
ADVERTISEMENT
1
2
3
4Completing the Square Using Algebra Tiles
2
2
(x+1)
2
(x+1)
+ 2
3
x+1
2
1. Use your calculator to graph the equation:
y
x
6
x
9
. Write the equation in vertex form.
=
+
+
2
2. Sketch algebra tiles to model the equation
y
x
6
x
9
.
=
+
+
4.5.1 Completing the Square – Algebra Tile Investigation
Recall the values of each of the algebra tiles. The value of the tile is its area. We will only be
working with positive (red tiles) representations of algebraic expressions.
e – Algebra Tile Investigation
(continued)
2
x
Tile
x Tile
Unit Tile
2
Area = x x = x
units
Area = 1 x = x units
Area = 1 1 = 1 unit
Area of the
Expression
mber
Length of
Unit Tiles
Square
Combining
Unit
the
Left Over (+)
Sketch of the Square
2
Our original equation was written in standard form,
. Since it is usually much easier to graph a
(Length)
Previous Two
2
y
ax
bx
c
Your Task:
=
+
+
With a partner, complete the following investigation.
es
Square
Borrowed (-)
1. Complete the table on the next two pages using algebra tiles.
Columns
parabola if the equation is in vertex form,
2
, often we try to rewrite the equation from quadratic
(
)
y
a
x
h
k
a) Represent each expression using the appropriate number of each of the algebra tiles.
=
−
+
b) Using the tiles you selected, try to create a square of tiles. When doing so, keep the
form into vertex form. We will use algebra tiles to help us understand why this procedure works.
following rules in mind:
2
You may only use one x
-tile in each square.
2
You must use all the x
and x-tiles. Unit tiles are the only ones that can be
(
)
When we are trying to write an equation in vertex form, we need to have a perfect square to make the
leftover or borrowed.
x − h
2
If you need more unit tiles to create a square you have to “borrow” them. The
part of the equation. When the quadratic equation we are given is not a perfect square, we arrange the parts to
number you borrow will be a negative quantity.
2
2
(x+1)
(x+1)
+ 2
3
x+1
You may create multiple squares, but they must have the same size
2
form a perfect square, adding what we need or keeping whatever extra pieces we may get. This activity will
help you discover how to start the process of forming the perfect square from what you are given.
2. After you have completed the table, answer the following questions:
2
a) What strategy did you use to place the x tiles around the x
tile?
e – Algebra Tile Investigation
(continued)
2
3. Create a partial square with algebra tiles to represent
x
+ x
2
______
.
+
Area of the
Expression
b) Can you create a square with an odd number of x tiles?
mber
Length of
Unit Tiles
22
Square
Combining
Unit
Sketch of the Square
the
Left Over (+)
a) How many unit tiles do you need to complete the square?
2
(Length)
Previous Two
c) What is the relationship between the value of “a” and the number of squares you
es
Square
Borrowed (-)
created?
Columns
d) What is the name of the form for the combined expression in the last column?
b) What are the dimensions of the completed square?
L =
W =
e) Why is “Completing the Square” an appropriate name for this procedure?
c) Replace c and ? with numbers to make the statement true:
2
2
(x+1)
(x+1)
+ 2
3
x+1
2
(
)
+ 2x + c = x + ?
2
2
x
Grade 11 U/C – Unit 4: Quadratic - Highs and Lows
21
+ 4x + ______ .
4. Create a partial square with algebra tiles to represent x
2
a) How many unit tiles do you need to complete the square?
b) What are the dimensions of the completed square?
L =
W =
c) Replace the c and ? with numbers to make the statement true:
(
)
+ 4x + c = x + ?
2
2
x
22
ADVERTISEMENT
0 votes
Related Articles
Related forms
Mcr3u Unit 1, Lesson 2 - A Review Of "completing The Square" And Vertex Form Worksheet With Answers - Saugeen District Secondary School
Education
Related Categories
Parent category: Education