Critical Values For Pearson'S R - Math Chart
ADVERTISEMENT
d.f.:
0.1
0.05
0.02
0.01
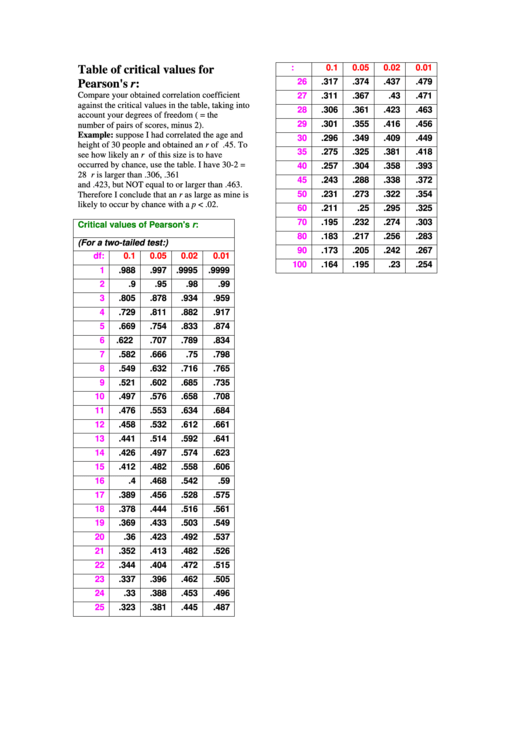
Table of critical values for
26
.317
.374
.437
.479
Pearson's r:
Compare your obtained correlation coefficient
27
.311
.367
.43
.471
against the critical values in the table, taking into
28
.306
.361
.423
.463
account your degrees of freedom (d.f.= the
29
.301
.355
.416
.456
number of pairs of scores, minus 2).
Example: suppose I had correlated the age and
30
.296
.349
.409
.449
height of 30 people and obtained an r of .45. To
35
.275
.325
.381
.418
see how likely an r of this size is to have
occurred by chance, use the table. I have 30-2 =
40
.257
.304
.358
.393
28 d.f. My obtained r is larger than .306, .361
45
.243
.288
.338
.372
and .423, but NOT equal to or larger than .463.
50
.231
.273
.322
.354
Therefore I conclude that an r as large as mine is
likely to occur by chance with a p < .02.
60
.211
.25
.295
.325
70
.195
.232
.274
.303
Critical values of Pearson's r:
80
.183
.217
.256
.283
(For a two-tailed test:)
90
.173
.205
.242
.267
df:
0.1
0.05
0.02
0.01
100
.164
.195
.23
.254
1
.988
.997
.9995
.9999
2
.9
.95
.98
.99
3
.805
.878
.934
.959
4
.729
.811
.882
.917
5
.669
.754
.833
.874
6
.622
.707
.789
.834
7
.582
.666
.75
.798
8
.549
.632
.716
.765
9
.521
.602
.685
.735
10
.497
.576
.658
.708
11
.476
.553
.634
.684
12
.458
.532
.612
.661
13
.441
.514
.592
.641
14
.426
.497
.574
.623
15
.412
.482
.558
.606
16
.4
.468
.542
.59
17
.389
.456
.528
.575
18
.378
.444
.516
.561
19
.369
.433
.503
.549
20
.36
.423
.492
.537
21
.352
.413
.482
.526
22
.344
.404
.472
.515
23
.337
.396
.462
.505
24
.33
.388
.453
.496
25
.323
.381
.445
.487
ADVERTISEMENT
0 votes
Related Articles
Related forms
Related Categories
Parent category: Education
 1
1








