Ph Chart - Chemistry Reference Sheet

ADVERTISEMENT

At 25°C, K
= 1.0×10
-14
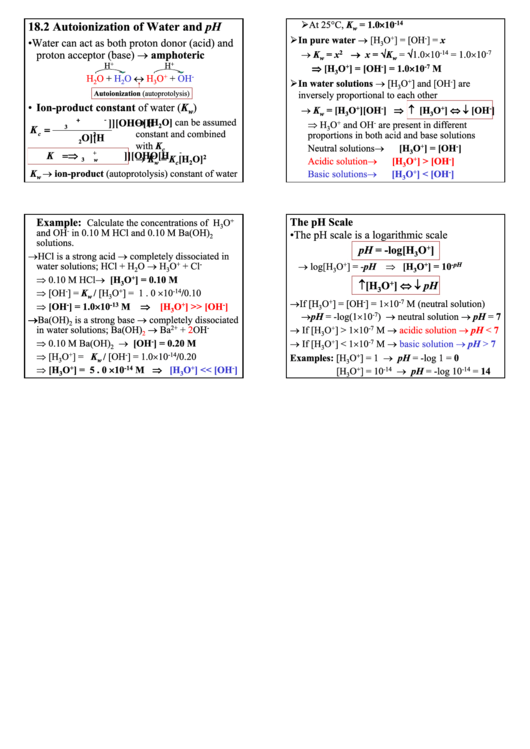
18.2 Autoionization of Water and pH
w
In pure water → [H
O
+
] = [OH
-
] = x
• Water can act as both proton donor (acid) and
3
proton acceptor (base) → amphoteric
→ K
→ x = √K
= √1.0×10
= x
2
-14
= 1.0×10
-7
w
w
H
+
H
+
⇒ [H
O
+
] = [OH
-
] = 1.0×10
-7
M
3
↔
+
-
H
O
+
H
O
H
O
+
OH
In water solutions → [H
O
+
] and [OH
-
] are
2
2
3
3
Autoionization (autoprotolysis)
inversely proportional to each other
• Ion-product constant of water (K
)
→ K
] ⇒ ↑[H
] ⇔ ↓[OH
= [H
O
+
][OH
-
O
+
-
]
w
w
3
3
+
→ [H
-
O] can be assumed
[H
O
][OH
]
⇒ H
O
+
and OH
-
are present in different
2
=
K
3
3
constant and combined
proportions in both acid and base solutions
c
2
[H
O]
2
with K
Neutral solutions →
[H
O
+
] = [OH
-
]
c
+
3
⇒
=
-
K
[H
O
][OH
]
→ K
= K
[H
O]
2
Acidic solution →
[H
O
+
] > [OH
-
]
w
3
w
c
2
3
→ ion-product (autoprotolysis) constant of water
→
K
Basic solutions
[H
O
+
] < [OH
-
]
w
3
The pH Scale
Example:
Calculate the concentrations of H
O
+
3
and OH
-
in 0.10 M HCl and 0.10 M Ba(OH)
• The pH scale is a logarithmic scale
2
solutions.
pH = -log[H
O
+
]
→HCl is a strong acid → completely dissociated in
3
O → H
→ log[H
] = -pH ⇒ [H
water solutions; HCl + H
O
+
+ Cl
-
O
+
O
+
] = 10
-pH
2
3
3
3
⇒ 0.10 M HCl → [H
+
O
] = 0.10 M
↑[H
] ⇔ ↓pH
O
+
3
3
⇒ [OH
-
] = K
/ [H
O
+
] = 1.0×10
-14
/0.10
w
3
→If [H
O
+
] = [OH
-
] = 1×10
-7
M (neutral solution)
⇒ [OH
M ⇒
-
] = 1.0×10
-13
[H
O
+
] >> [OH
-
]
3
3
→pH = -log(1×10
) → neutral solution → pH = 7
-7
→Ba(OH)
is a strong base → completely dissociated
2
→ Ba
→ If [H
M →
acidic solution → pH < 7
in water solutions; Ba(OH)
2+
+ 2OH
-
O
+
] > 1×10
-7
2
3
⇒ 0.10 M Ba(OH)
→ [OH
→ If [H
M →
basic solution → pH > 7
-
] = 0.20 M
O
+
] < 1×10
-7
2
3
⇒ [H
O
+
] = K
/ [OH
-
] = 1.0×10
-14
/0.20
] = 1 → pH = -log 1 = 0
Examples: [H
O
+
3
w
3
⇒ [H
M ⇒
O
+
] = 5.0×10
-14
[H
O
+
] << [OH
-
]
→ pH = -log 10
[H
O
+
] = 10
-14
-14
= 14
3
3
3

ADVERTISEMENT

00 votes

Related Articles

Top 20 Happy Easter Cards And Gift Tags To Download For Free
Top Ten Easter Arts And Crafts Activities For Your Kids
Free Easter Coloring Pages And Easter Printables For Your Kids

Related forms

Chemistry Reference Sheet - Cations Flow Chart Chemistry Reference Sheet - Cations Flow Chart Education
Chemistry Reference Sheet Periodic Table Of The Elements Chemistry Reference Sheet Periodic Table Of The Elements Education
Chemistry Reference Sheet Chemistry Reference Sheet Education
Chemistry Reference Sheet - Mr.torre Chemistry Reference Sheet - Mr.torre Education
Volume, Weight Conversions, Sulfur Dioxide And Ph Chemistry Reference Sheet Volume, Weight Conversions, Sulfur Dioxide And Ph Chemistry Reference Sheet Education
Glossary (chemistry Reference Sheet) Glossary (chemistry Reference Sheet) Education
Ap Chemistry Reference Sheet Ap Chemistry Reference Sheet Education
Chemistry Math Reference Sheet Chemistry Math Reference Sheet Education
Chemistry Reference Table Chemistry Reference Table Education
Chemistry Reference Materials Chemistry Reference Materials Education

Related Categories

Parent category: Education
Download
Go
Page of 3