Core Mathematics Worksheet
ADVERTISEMENT
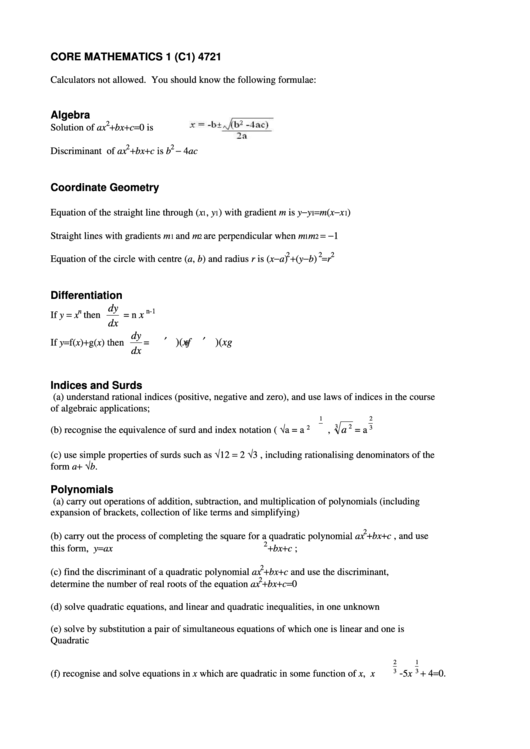
CORE MATHEMATICS 1 (C1) 4721
Calculators not allowed. You should know the following formulae:
Algebra
2
+bx+c=0 is
Solution of ax
2
+bx+c is b
2
− 4ac
Discriminant of ax
Coordinate Geometry
=m(x−x
Equation of the straight line through (x
, y
) with gradient m is y−y
)
1
1
1
1
= −1
Straight lines with gradients m
and m
are perpendicular when m
m
1
2
1
2
2
+(y−b)
2
=r
2
Equation of the circle with centre (a, b) and radius r is (x−a)
Differentiation
dy
If y = x
n
n-1
x
then
= n
dx
dy
f ′
g ′
(x
)
(x
)
If y=f(x)+g(x) then
=
+
dx
Indices and Surds
(a) understand rational indices (positive, negative and zero), and use laws of indices in the course
of algebraic applications;
1
2
3
2
(b) recognise the equivalence of surd and index notation (e.g. √a = a
2
3
a
,
= a
(c) use simple properties of surds such as √12 = 2 √3 , including rationalising denominators of the
form a+ √b.
Polynomials
(a) carry out operations of addition, subtraction, and multiplication of polynomials (including
expansion of brackets, collection of like terms and simplifying)
2
+bx+c , and use
(b) carry out the process of completing the square for a quadratic polynomial ax
2
+bx+c ;
this form, e.g. to locate the vertex of the graph of y=ax
+bx+c and use the discriminant, e.g. to
2
(c) find the discriminant of a quadratic polynomial ax
2
+bx+c=0
determine the number of real roots of the equation ax
(d) solve quadratic equations, and linear and quadratic inequalities, in one unknown
(e) solve by substitution a pair of simultaneous equations of which one is linear and one is
Quadratic
2
1
+ 4=0.
3
3
-
(f) recognise and solve equations in x which are quadratic in some function of x, e.g. x
5x
ADVERTISEMENT
0 votes
Related Articles
Related forms
Related Categories
Parent category: Education
 1
1 2
2








